Bubble Chamber Beam Test September 2015

Bubble Chamber Beam Test

• Chamber is filled with natural N₂O

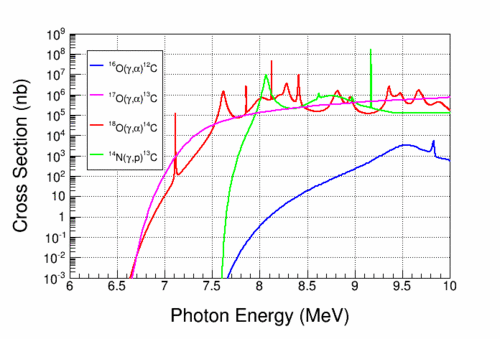
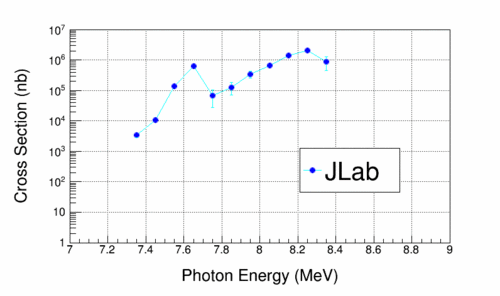
• Cross sections:

The cross sections are shown in the following figure:

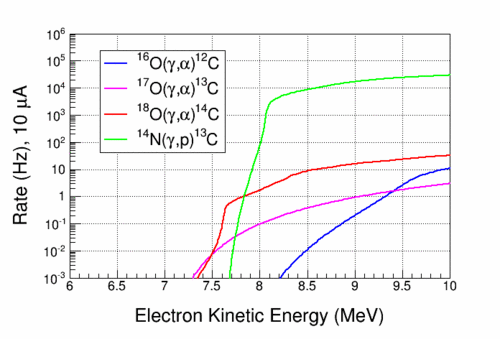
• Expected rates:

The expected rates are calculated with 3 cm cell thickness and thin radiator.

• The expected rates are shown in the following figure:

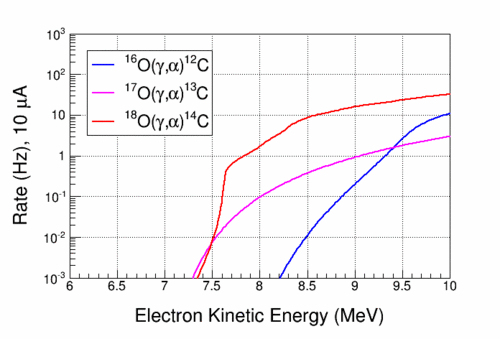
• The expected rates from oxygen isotopes are shown in the following figure:

---

• Run Plan:

• Check if Bubble Chamber is working properly:

1. Start with K.E. of 7.7 MeV
2. Determine an initial pressure and temperature with no beam
3. Measure bubble rate. The expected rate is 1 bubble per sec at 10 µA
4. Measure rate vs beam current (0.01 – 10 µA)

• Suppression of ¹⁴N(γ,p)¹³C events:

1. Start with beam at K.E. 7.0 MeV
2. Increase beam K.E. by 0.2 MeV steps all the way to 8.6 MeV
3. Adjust beam current to maintain a bubble rate of few per minute
4. Reproduce the overall rate shown above
5. At K.E. of 8.6 MeV, increase the chamber threshold by increasing the pressure. Suppress the ¹⁴N(γ,p)¹³C events.
6. Once ¹⁴N(γ,p)¹³C is suppressed, the rate should drop by three orders of magnitude

• Measuring rates from ¹⁸O(γ,α)¹⁴C:

1. Now the chamber is at the new pressure
2. Start with beam at K.E. 7.2 MeV
3. Increase beam K.E. by 0.2 MeV steps all the way to 9.2 MeV
4. Adjust beam current to maintain a bubble rate of few per minute
5. Reproduce the overall rate from oxygen isotopes shown above
6. Note the change in slope of rates vs. beam K.E. and the sharp kink around 7.6 MeV

• Measuring ¹⁸O(γ,α)¹⁴C cross section:

1. Start with beam at K.E. 7.4 MeV
2. Increase beam K.E. by 0.1 MeV steps all the way to 8.4 MeV
3. Adjust beam current to maintain a bubble rate of few per minute
4. Perform Penfold-Leiss unfolding

Beam Kinetic Energy (MeV)	Beam Current (µA)	Time (hour)
7.4	10	10
7.5	10	10
7.6	10	5
7.7	2	5
7.8	2	5
7.9	1	5
8.0	1	5
8.1	0.8	5
8.2	0.4	5
8.3	0.2	5
8.4	0.2	5

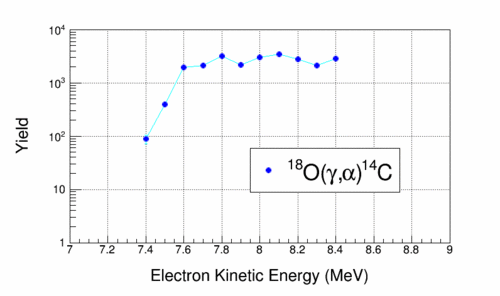
The expected yield is shown in the following figure:

The Penfold-Leiss unfolded cross section is shown in the following figure: